Algunos enlaces y links de Filtros Activos parte 2

http://cnyack.homestead.com/files/modulation/modfmplla.htm

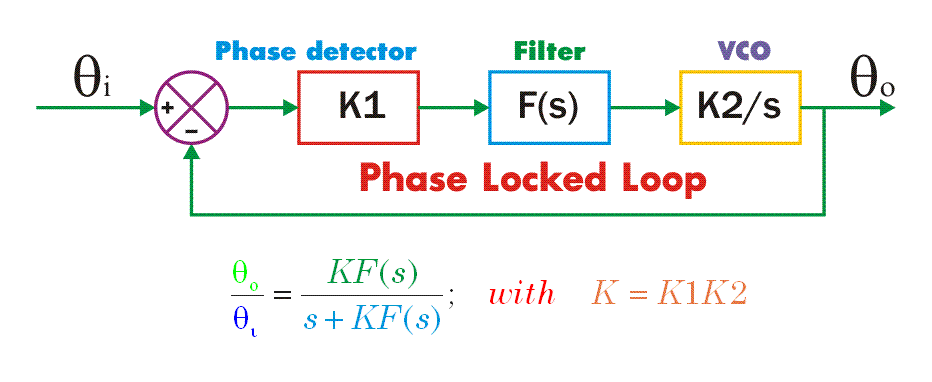
Phase Locked Loop and FM Demodulation, Active Filters.

Cuthbert Nyack

---

A block diagram of the phase Locked Loop is shown above. There are 3 main components in the loop. First is a Phase Detector shown as the subtractor and amplifier with gain K1. Second is a Loop Filter with transfer function F(s) and third is a Voltage Controlled Oscillator with transfer function K2/s. Some possibilities for filters using active circuits are shown above. Compared with their passive counterparts, these filters are of type 1, ie their gain becomes ~ 1/(Tp1 s) at low frequencies. These filters can increase the gain of the loop and reduce loop rise time. Being Type 1, they can eliminite errors for ramp phase inputs. Fig 1 has constant gain of Tz/Tp at high frequencies. Fig 2 has gain of Tz/(Tp1 Tp2 s) at high frequencies and can be used for higher attenuation of high frequency components coming from the phase detector.
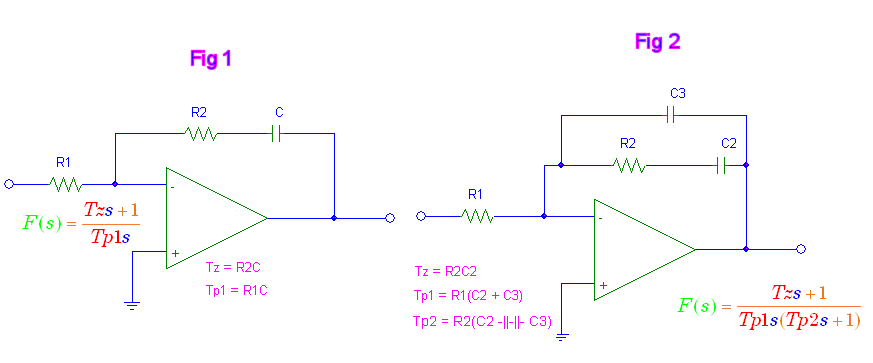
There are very limited restrictions on Tz, Tp1 and Tp2 in the applet and CAUTION is required when using high values of Tz and low values of Tp1 and Tp2. These should be related to physically realistic values. Tp2 must be less than Tz.

Fn = 62 shows the frequency response of the filters.
Fn = 63 to 66 show special cases of Fn = 62.

---

Some of the properties of loops which use the filter of Fig 1 are shown by Fn = 1 to 30.
Fn = 1 shows the phase step, frequency impulse response.
Fn = 2 to 5 show special cases of Fn = 1.
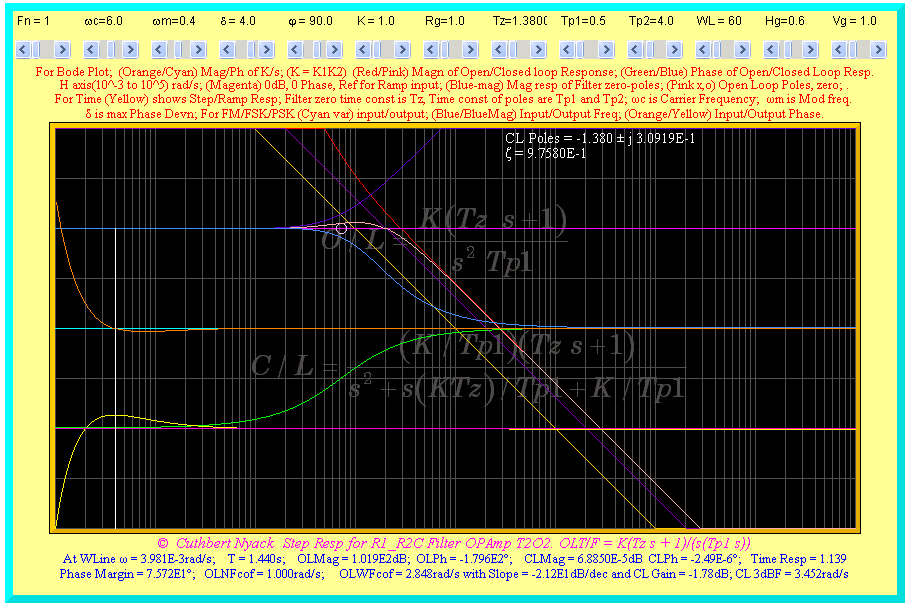
Image below shows a case of Fn = 1, Transient Phase response is in yellow and frequency response is in dark orange. In this case Tp1 changes both the risetime and the damping and Tz can be used to damp out the transient oscillations.

---

Fn = 6 shows the transient behaviour, Bode plot and Root Locus plot. Fn = 7 to 12 show special cases of Fn = 6.

---

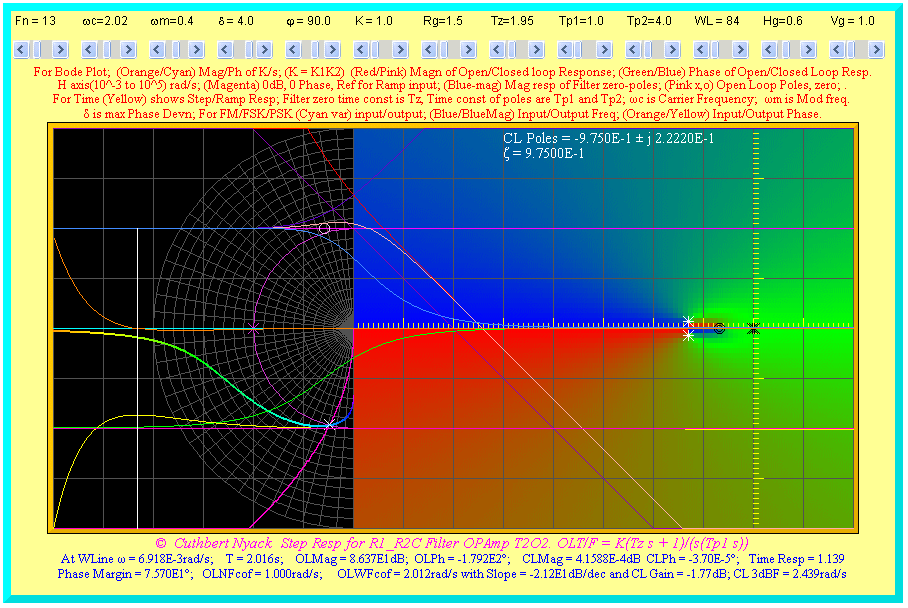
Fn = 13 shows the Bode plot, Nyquist plot, Root Locus and transient behaviour.
Fn = 14 and 15 show special cases of Fn = 13.
A example of Fn = 13 is shown in the image below. The standard Nyquist plot is the heavier pink magenta line and the multicolored line is a "compressed" version of the Nyquist plot which shows the behaviour over a wider range of frequencies. The white x is moved by wc and shows the point where the magnitude of the gain is 1. This is the point at which the phase margin is calculated.

---

Fn = 16 shows the ramp response for this system.
Fn = 17 and 18 are special cases of Fn = 16. An example of the ramp response for this system is shown in the image below. Ramp input is in magenta, phase ramp output is in yellow and frequency step output is in dark orange.
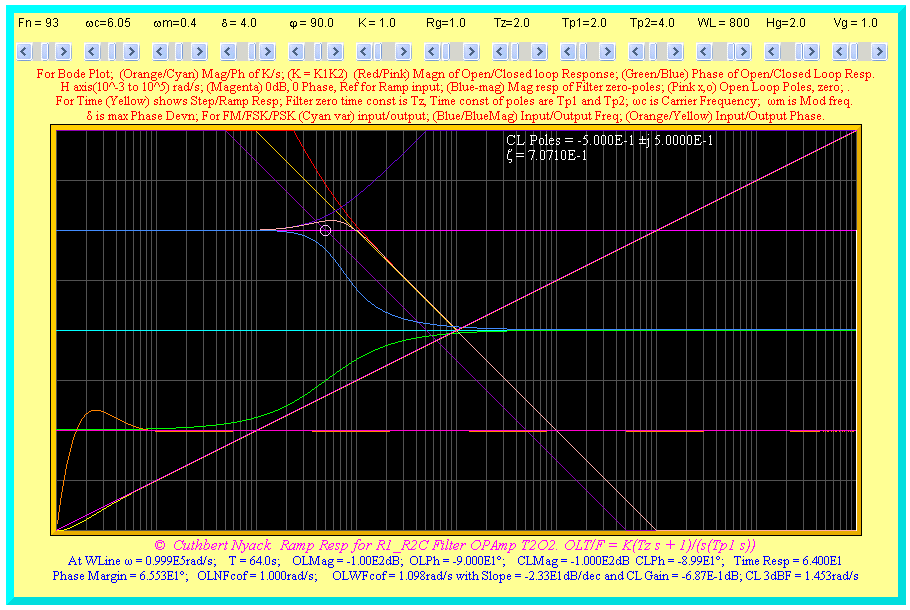
Since this is a Type 2 system, then the phase error to a ramp input is zero. In the first blue line we see that at t = 64, the input is 64 and the response is also 64.

---

Fn = 19 shows a parabolic phase input.
Fn = 20 and 21 show special cases of Fn = 19.
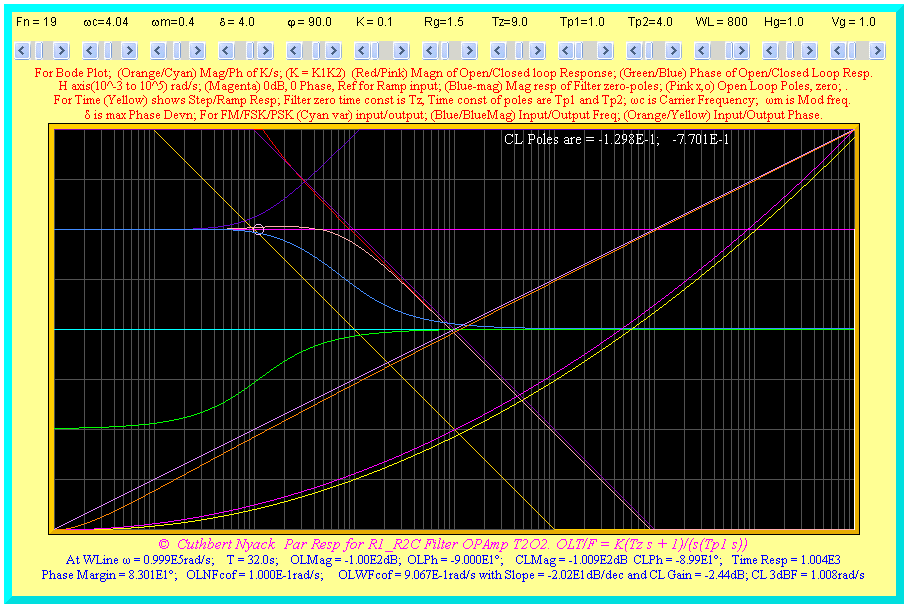
An example of the parabolic response is shown in the image below. The phase parabolic error which is the difference between the magenta and yellow lines is constant in time while the frequency ramp error tends to zero.

---

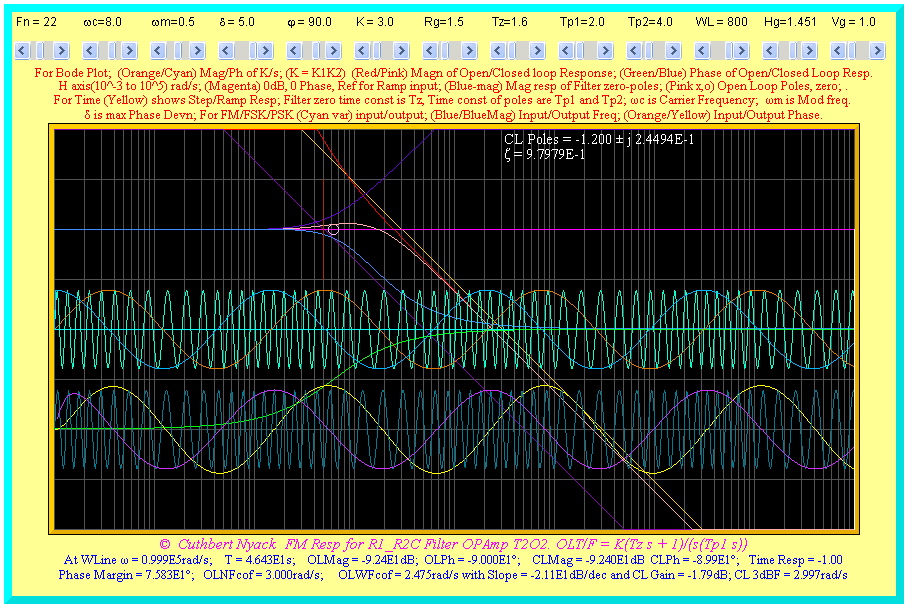
Fn = 22 shows the FM response.
Fn = 23 and 24 show special cases of Fn = 22.
Fn = 25 shows the FSK response with 2 frequencies.
Fn = 26 and 27 show special cases of Fn = 25.
Fn = 28 shows the PSK response with 2 phases.
Fn = 29 and 30 show special cases of Fn = 28.
Note that the PSK response contains a frequency impulse everytime the phase changes.
Image below show a case of the FM response.

---

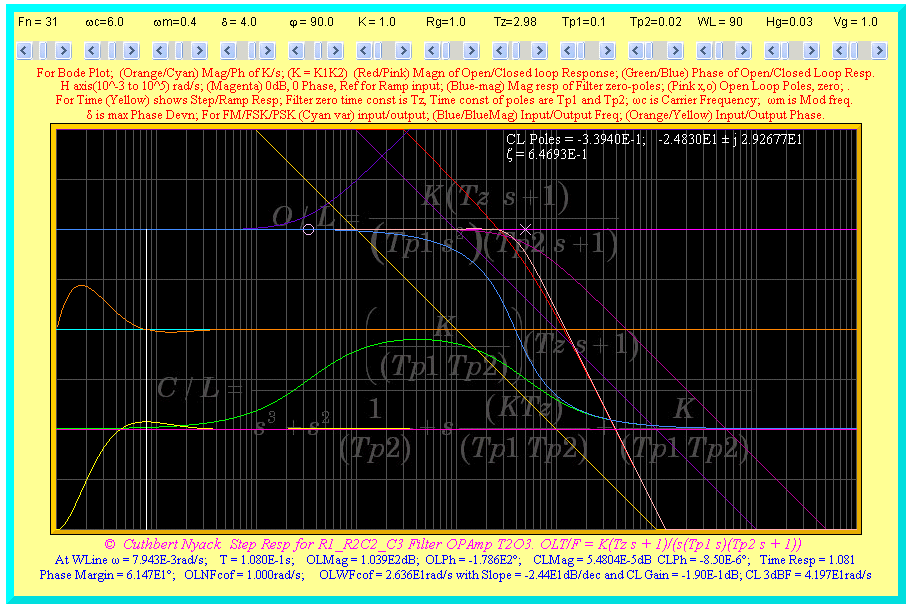
Fn = 31 to 61 show the corresponding cases for the Filter of Fig 3. Since this is an active filter, it can increase the 3dB bandwidth and reduce the risetime as shown below.

---

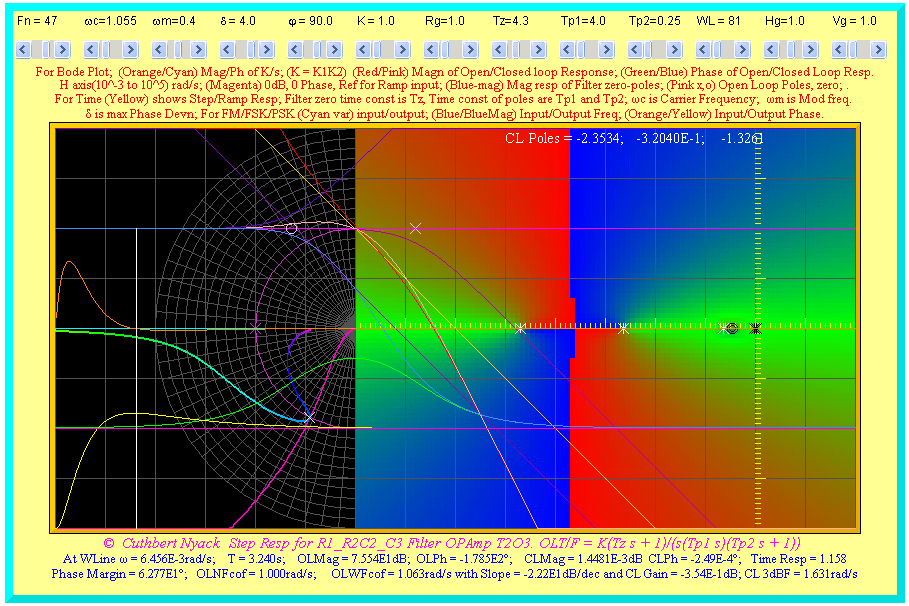
An example showing the Nyquist plot is shown below. The compressed version of the Nyquist plot is convenient for adjusting the frequency response so the minimum phase delay occurs at the crossover frequency.

---

Fn = 62 shows the frequency response of the filters.
Fn = 63 to 66 show special cases of Fn = 62.

---

Applet should appear below.

---

Return to main page
Return to page index

---

COPYRIGHT © 2012 Cuthbert Nyack.